LIMBO

Cards

Deal five cards each from a 52-card pack and stack the rest face up.

Object

Play begins with a count of 101, and as each card is played it reduces the count. The count may not be reduced below zero, and whoever plays the last card wins the deal.

Doubling

Before playing the first card the leader may announce "Double", in which case the winner's eventual score will be doubled. The second player may then either double, if the first does not, or redouble the leader's double, in which case the winner's score will be quadrupled.

Play

Dealer takes the top card of the stock, places it face up on the table, deducts its value from 101, and announces the new total. If the starter is a face card, it counts as zero and leaves the count at 101. Then each in turn, starting with non-dealer, plays a card face up on the previous one and announces the new count. If you play a numeral (from Two = 2 to Ten = 10) its value is deducted from the count, unless it divides without remainder, in which case it must be divided, not subtracted.

Examples: Dealer turns a Five as the start card and announces "96". Non-dealer might then play a Seven and announce "89" (by deduction), or a Six and announce "16" (by division), but may not deduct 6 to make 90 (because division is possible and therefore mandatory). If the count has more than one divisor you may freely choose between them. Thus if the first card is 5, reducing the count to 96, and you hold 2,3,4,6,8, you may play any of them, to make the count respectively 48, 22, 24, 16, or 12.

Aces

An Ace counts either 1 or 11, as you choose; but as you must always reduce the count you may only deduct it, not use it to divide by one. (Most games will end at a count of 1, but if the other player has an Ace they can - and indeed must - then deduct it to make zero.)

Face cards

Face cards have special properties, which may be summarised as "Jack repeats, Queen reverses, King divides".

• Jack. A Jack played repeats the same value as the previous card, or assumes the same power if the previous card was a King or Queen.
• Queen. A Queen reverses the digits of the current count. For example, if the count is 96, playing a Queen converts it to 69. However, you may only play a Queen if reversing the digits reduces the count. So, for example, a Queen is unplayable on counts such as 69, or even 66. (But note that a Queen played to a count of 60, for example, makes it 6.)
• King. A King divides by any desired number higher than 1 and lower than the current, provided that it leaves no remainder.

End of play

The hand ends when one player plays their last card or is unable to play without going below zero. If you play your last card you may, if possible, continue by playing the top card of the face-up stock, provided that it is legally playable. If not, your opponent may continue playing alone for as long as possible in order to increase their own score and reduce yours.

 

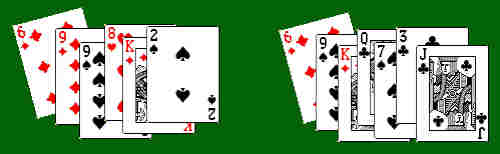
Two games played with the same hands after a Six is turned to reduce the start count to 95. In the first game, the cards played are -9 = 86, -9 = 77, -8 = 69, ÷K = 3 (dividing by 23), 2 = 1. Thus the first player wins with a score of 60 to the other's 10. In the second game, the play is -9 = 86, ÷K = 43 (dividing by 2), &Q = 34 (reversing digits), -7 = 27, ÷3 = 9, &J = 3 (repeating the previous 3 and necessarily dividing). Now the second player wins by 70 to 30.

Score

Whoever played the last card is the winner and scores 10 times the number of cards played (counting the starter), while the loser scores 10 times the value of the final count (which may be zero). Any doubling or redoubling that may have taken place is applied only to the winner's score, not the loser's. The winner then deals to the next hand. It seems unnecessary to shuffle: you can just keep playing through the pack to the end of the game.

Game

Play up to 200 points, or any other agreed target, or for any number of deals, or until you get fed up with it.

Beat that!